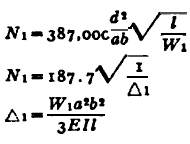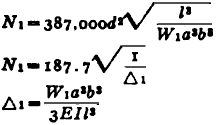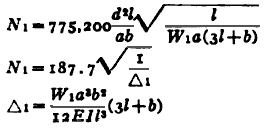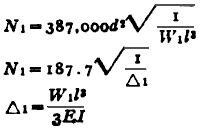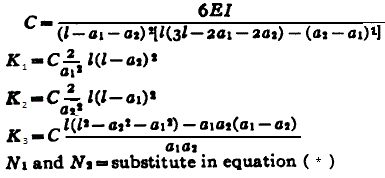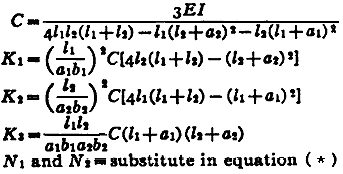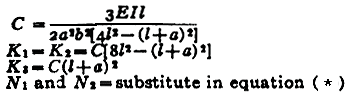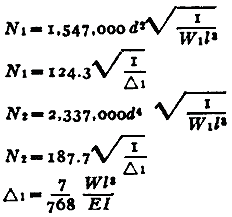Critical Speeds of Rotating Shafts with Single Loads Equations and Calculators

Bearings Application Knowledge Menu
Critical Speeds of Rotating Shafts or Mass Review

Critical Speeds of Rotating Shafts with Single Loads: When calculating critical speeds, the weight or mass of the rotating cylinder or shaft is assumed to be zero or add 1/2 to 2/3 of the rotating shaft to the load mass. Keep in mind that a shaft with more than one load or distributed loads may have an infinite number of critical speeds. Typically, the first critical speed is most important for your design.

NOTE: All Calculators require a Premium Membership to access.

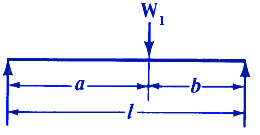
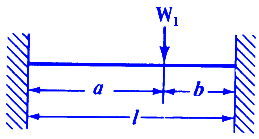
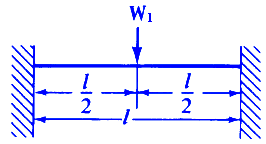
Load Configuration	Equation
	Open: Critical Speeds Rotating Shaft Variable Load location Calculator
	Open: Critical Speeds Rotating Shaft Load at Center Calculator
	Open: Critical Speeds Rotating Shaft Fixed Ends Calculator
	Open: Critical Speeds Rotating Shaft Fixed Ends Central Load Applied Calculator
	Open: Critical Speeds Rotating Shaft One Fixed End Calculator
	Open: Critical Speeds Rotating Shaft Fixed End Cantilevered Calculator
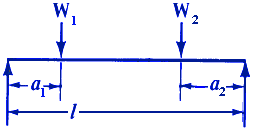
Two Concentrated Loads General Equation *
	Open: Critical Speeds Rotating Shaft fixed Ends Two Loads Calculator
	Open: Critical Speeds Rotating Shaft Fixed Ends Two Identical Loads Calculator
	Open: Critical Speeds Rotating Shaft Fixed Ends Two Identical Loads Calculator
	Open: Critical Speeds Rotating Shaft Fixed Ends Identical Load Distances Calculator
	Open: Critical Speeds Rotating Shaft Fixed Ends Identical Load Distances Calculator
	Open: Critical Speeds Rotating Shaft Fixed Ends Identical Load Distances Calculator

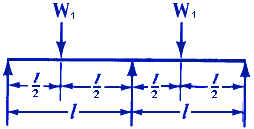Where:

N1	= first critical speed, RPM
N2	= second critical speed, RPM
Δ1	= static deflection, (in, m) at W1 if shaft is horizontal
Δ2	= static deflection, (in, m) at W2 if shaft is horizontal
E	= modulus of elasticity (young's modulus), (psi, N/m2)
d	= diameter of shaft, (inches, m)
W1	= load applied to shaft, (lbs, Kg)
I	= Area Moment of Inertia , (in4, m4)
l	= distance between centers of bearings, (inches, m)
a	= distance from bearings to load, (inches, m)
b	= distance from bearings to load, (inches, m)

Related Resources:

• Critical Speeds of Rotating Shafts with Distributed Loads Equations
• Critical Speeds of Rotating Shafts Review